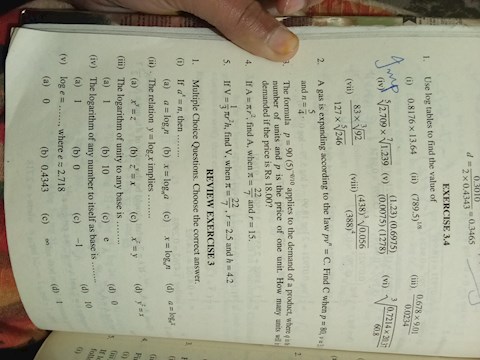
Government School 9th Grade Exercise 3.4

1. Solve the following equations:
a) 3x + 5 = 17
b) 2y - 7 = 11
c) 4z + 8 = 20

2. Simplify the following expressions:
a) 2(3x + 4) - 5x
b) 5(2y - 3) + 7y
c) 3(4z + 2) - 2(2z + 3)

3. Solve the following inequalities:
a) 2x + 3 < 11
b) 4y - 5 > 7
c) 3z + 6 ≤ 15

4. Find the value of x in the equation:
5x - 2 = 18

5. Solve the following word problems:
a) If a number is increased by 8, the result is 20. What is the number?
b) The sum of two consecutive even numbers is 46. Find the numbers.
c) A rectangle has a length of 12 cm and a width of x cm. If the perimeter is 40 cm, find the value of x.

Exercise 3.4: Solving Quadratics by Completing the Square

Instructions:

1. Solve each quadratic equation by completing the square.
2. Show all your steps in detail.

Questions:

1. x² - 8x + 15 = 0
2. 2x² - 12x + 11 = 0
3. x² + 6x - 7 = 0
4. 3x² + 18x - 10 = 0

Solutions:

1. x² - 8x + 15 = 0

- Subtract 15 from both sides: x² - 8x = -15
- Divide by 4 to complete the square of the left side: (x - 4)² = -4
- Take the square root of both sides: x - 4 = ±√(-4) = ±2i
- Solve for x: x = 4 ± 2i

2. 2x² - 12x + 11 = 0

- Divide by 2 to make the coefficient of x² equal to 1: x² - 6x + 5.5 = 0
- Subtract 5.5 from both sides: x² - 6x = -5.5
- Divide by 3 to complete the square of the left side: (x - 3)² = -1.833
- Take the square root of both sides: x - 3 = ±√(-1.833) = ±1.354i
- Solve for x: x = 3 ± 1.354i

3. x² + 6x - 7 = 0

- Add 7 to both sides: x² + 6x = 7
- Divide by 2 to complete the square of the left side: (x + 3)² = 16
- Take the square root of both sides: x + 3 = ±√16 = ±4
- Solve for x: x = -3 ± 4

4. 3x² + 18x - 10 = 0

- Divide by 3 to make the coefficient of x² equal to 1: x² + 6x - 10/3 = 0
- Subtract 10/3 from both sides: x² + 6x = 10/3
- Divide by 3 to complete the square of the left side: (x + 3)² = 34/3
- Take the square root of both sides: x + 3 = ±√(34/3) = ±2.828
- Solve for x: x = -3 ± 2.828

Question 1